A Tusi Couple, and they are not even dating!

This is done in GeoGebra, but the setting is old.
Google "Tusi couple" and follow the evidence.
Explore.

 

Is this the best NYSED can do? (Turkey continued..)

The "commentary" following the problem referred to in How do you cook a turkey? (my blog post from yesterday) states the following (page 27 here):

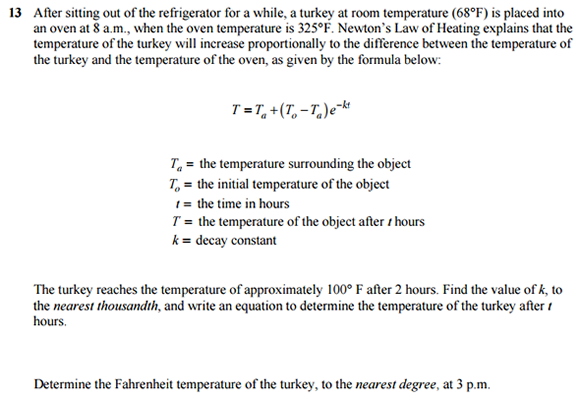
This question measures A-CED.A because students must create an exponential
equation and use it to solve problems.

I beg to differ: the exponential equation is not created by the student, but handed to them and credited to Newton.

Once it is recognized that this problem does NOT address the part of the common core standards it claims to, its reason for existence in this setting is gone.

This problem is connected to "Mathematical Practice(s) 1 and 4 (see page 27 here).  Mathematics practice 1 is stated here (italics are mine):

MP 1 - Make sense of problems and persevere in solving them.

Mathematically proficient students start by explaining to themselves the meaning of a problem and looking for entry points to its solution. They analyze givens, constraints, relationships, and goals. They make conjectures about the form and meaning of the solution and plan a solution pathway rather than simply jumping into a solution attempt. They consider analogous problems, and try special cases and simpler forms of the original problem in order to gain insight into its solution. They monitor and evaluate their progress and change course if necessary. Older students might, depending on the context of the problem, transform algebraic expressions or change the viewing window on their graphing calculator to get the information they need. Mathematically proficient students can explain correspondences between equations, verbal descriptions, tables, and graphs or draw diagrams of important features and relationships, graph data, and search for regularity or trends. Younger students might rely on using concrete objects or pictures to help conceptualize and solve a problem. Mathematically proficient students check their answers to problems using a different method, and they continually ask themselves, “Does this make sense?” They can understand the approaches of others to solving complex problems and identify correspondences between different approaches. 

This problem expects students to jump in and attempt a solution using the equation they are given.

Mathematical practice 4 is here:

MP 4 - Model with mathematics.

Mathematically proficient students can apply the mathematics they know to solve problems arising in everyday life, society, and the workplace. In early grades, this might be as simple as writing an addition equation to describe a situation. In middle grades, a student might apply proportional reasoning to plan a school event or analyze a problem in the community. By high school, a student might use geometry to solve a design problem or use a function to describe how one quantity of interest depends on another. Mathematically proficient students who can apply what they know are comfortable making assumptions and approximations to simplify a complicated situation, realizing that these may need revision later. They are able to identify important quantities in a practical situation and map their relationships using such tools as diagrams, two-way tables, graphs, flowcharts and formulas. They can analyze those relationships mathematically to draw conclusions. They routinely interpret their mathematical results in the context of the situation and reflect on whether the results make sense, possibly improving the model if it has not served its purpose.

This problem expects, almost requires, that the student do no modelling whatsoever. The modelling has already been done by Isaac Newton.

Come on NYSED, fix this.

How do you cook a Turkey?

The question below is taken from the New York State Common Core Sample Questions: Regents Examination in Algebra II (Common Core) Fall 2015. The complete file can be found  here.

This question makes reference to Newton's Law of Heating. Unless you are familiar with this law, I suggest you follow this link.

Now my concern: what is this law doing in an Algebra II exam of any kind?

Here we are at exam time, and we throw at our students a concept they are unfamiliar with in such a way that we believe we are only asking them this:

a) Solve for k: \[100 = 325 + \left( {68 - 325} \right){e^{ - 2k}}\]

 b) Use your value for k to solve; \[T = 325 + \left( {68 - 325} \right){e^{ - 7k}}\]

Everything else in this question, other than the use of these two items,  involves the careful reading of a passage in the realm of thermodynamics, interpreting the contents carefully, and properly substituting values into s formulas handed out freely.  All three skills are worthy, but should be tested in a familiar realm. The writer of the question knows that "object" here refers to the turkey, but would that be obvious to the novice, reading such a scenario for the first time?

This question also includes some either dangerous or misleading (or even false) information. 

The most important is that the time needed to bring a refrigerated turkey to room temperature would be far too long, allowing for growth of salmonella among other hazards. The typical cook may wait for the surface of the turkey to feel close to room temperature, but never waits for the whole bird to warm up.

Second, Newton's law of Cooling (the proper name of the law) is based on the object having a uniform temperature. In any other situation the law provides nothing more than an approximation. As noted above, that is not the case when we cook a turkey. (Ever notice that the temperature can read differently when the thermometer is moved to a different location in the turkey?)

Thirdly, take note of this, a typical chart (from Foster Farms)

The turkey in this problem is cooked for 7 hours (who knows, maybe more?). This is either a huge turkey in an industrial oven, or someone who likes their turkey as little on the burnt side.

No matter how I slice it (no pun intended), this problem comes across to me as being totally out of place in an Algebra II exam. It amounts to a "cookbook" problem, (pun unintended) the likes of which have no place even near an end-of-the-year Regents exam in Algebra II.

GeoGebra as a Presentation Tool

I made this this morning just to be an example of how GeoGebra can be used to create dynamic presentations for teachers.
It is pretty simple to follow and requires no knowledge of GeoGebra. It can be downloaded from GeoGebratube here.
Enjoy!

If only... (updated)

What I could have done in the classroom if I had had GeoGebra, a smartboard, and a tablet/laptop of every student. I would toss the textbook, and go.

Here is a sketch easy to create, but full of mathematical questions.
I have included the option of showing some parametric info just as a "spur".

The blue point, the center of the blue circle, rotates on the green circle.
The red point rotates on the blue circle. The red and blue points complete one rotation at the same time.
Does this generate an ellipse?

Start with parametric equations as above.
\(\begin{array}{l}x = n\cos ( - \theta ) + m\cos (\theta )\\y = n\sin ( - \theta ) + m\sin (\theta )\end{array}\)

Use negative angle identities.
\(\begin{array}{l}x = n\cos (\theta ) + m\cos (\theta )\\y =  - n\sin (\theta ) + m\sin (\theta )\end{array}\)

Factor
\(\begin{array}{l}x = \left( {n + m} \right)\cos (\theta )\\y = \left( { - m + n} \right)\sin (\theta )\end{array}\)

Rewrite:
\(\begin{array}{l}\frac{x}{{n + m}} = \cos (\theta )\\\frac{y}{{m - n}} = \sin (\theta )\end{array}\)

Square and add
\({\left( {\frac{x}{{n + m}}} \right)^2} + {\left( {\frac{y}{{m - n}}} \right)^2} = {\cos ^2}(\theta ) + {\sin ^2}(\theta )\)

Use Pythagorean identity
\({\left( {\frac{x}{{n + m}}} \right)^2} + {\left( {\frac{y}{{m - n}}} \right)^2} = 1\)

Rewrite
\(\frac{{{x^2}}}{{{{\left( {n + m} \right)}^2}}} + \frac{{{y^2}}}{{{{\left( {m - n} \right)}^2}}} = 1\)

We now have a standard equation for an ellipse.

Birth of an Ellipse (Revisited)

As we begin the new year, I am revisiting one of my past GeoGebra creations. This shows how an ellipse results from tracking the midpoint as the endpoints of a segment rotate around two circles with the same center. The points rotate in opposite directions, but complete an orbit in the same amount of time. 

This is just one of my examples intending to show how topics generally left for later in high school can be introduced much earlier. This example reinforces concepts such as circle, rotation, segment, and midpoint while generating an ellipse. The equations shown in the process can be eliminated or hidden. They were included for those who wish to connect this with higher concepts in Algebra II or later.

This is also my first post to be shared with Facebook, which I have joined, at least for now.  Consider this a test!

normal probability cumulative density function

If the title of this post seems awkward, imagine my feelings when I found it on page 36 of New York State's Algebra II Fall Sampler from Fall 2015.

I encourage you to look at that link, and keep in mind that just a few minutes ago I Googled that phrase (in quotes) and got 3 results, with the Fall Sampler being the second in the list. Here it is. ( I clicked the "If you like" at the bottom, and picked up one more link.)

The Common Core states that students should be able to "Use calculators, spreadsheets, and tables to estimate areas under the normal curve."

The area under a curve is a whole subject in and of itself (part of Calculus), and its discovery (or invention?) generally begins with rectangle approximations, trapezoidal approximations, limits, continuity, etc. Are these all part of Algebra II? I know that the process of approximation of the area of a portion of the Cartesian plane bounded above and below by continuous functions of x is very highly programmable. A lot of mathematics is involved in creating such a program. Expecting high school students to use such a program while remaining ignorant of the mathematics involved in its creation seems to be a disservice to those students.

The whole business about Common Core seemed predicated on understanding mathematics. Does someone who has mastered the art of pushing buttons on a calculator understand this concept? And whose idea was it to base a high school sample question on "normal probability cumulative density function".